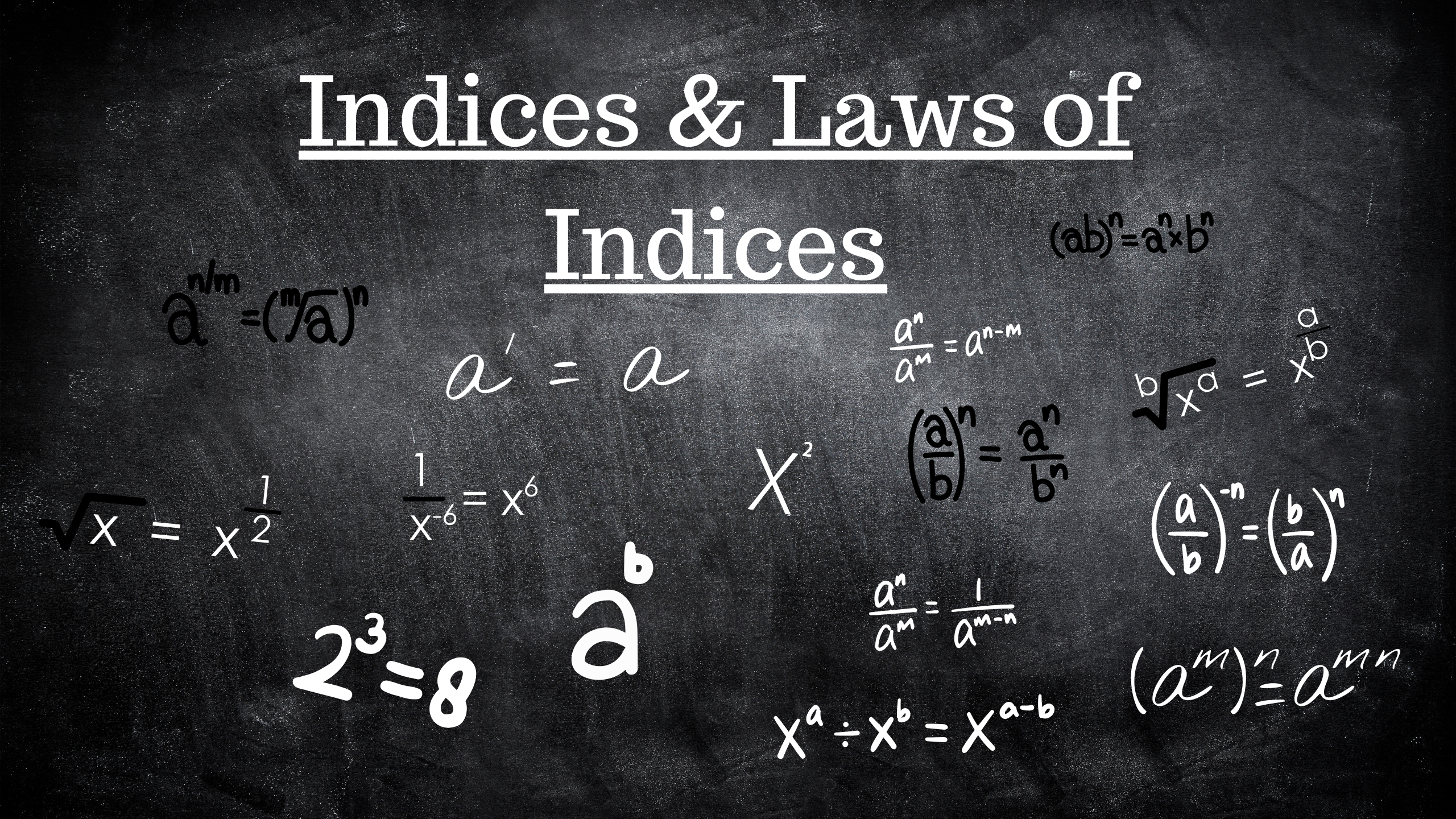
Indices, also known as exponents or powers, are a fundamental concept in mathematics that play a crucial role in simplifying and manipulating expressions. In this article we will delve into the world of indices, exploring what they are, their significance, and the laws that govern their operations.

What are Indices?

Indices are small numbers written above and to the right of another number, often called the base. This small number indicates the power to which the base is raised.

For example, in the expression , 2 is the base, and 3 is the index. The result is obtained by multiplying 2 by itself three times: .

Indices provide a concise way of expressing repeated multiplication. They are not limited to integers and can be fractions or even irrational numbers. Let’s take a look at the laws of indices and their formulas.


Laws of Indices

Understanding the laws that govern indices is key to simplifying expressions and solving equations efficiently. Let’s explore some fundamental laws of indices:

1. Product Law

The product law states that when you multiply two expressions with the same base, you can add their indices.
                                              

   

For example:

   

Here, the product law allows us to combine the bases and add the exponents.

2. Quotient Law

The quotient law dictates that when you divide two expressions with the same base, you subtract the indices.
                                             

   

For example:
  

   

The quotient law simplifies the division of terms with common bases.

3. Power Law

The power law states that when you raise an already exponentiated expression to another power, you multiply the indices.
                                                     

   

For example:
                                            

   

This law allows us to consolidate exponents efficiently.

4. Zero Exponent Law

Any nonzero number raised to the power of zero is always 1.
                                                          

   

For example:
                                                         

   

The zero exponent law simplifies expressions where the exponent is zero.

5. Negative Exponent Law
A negative exponent indicates the reciprocal of the base raised to the positive exponent.
                                                  

   

For example:
                                      

   

The negative exponent law allows us to express reciprocals conveniently.

Applications of Indices

Understanding indices is not merely an exercise in mathematical manipulation; it has practical applications across different scientific and engineering fields. Consider compound interest, where the interest earned in each period is calculated using indices. Additionally, indices are prevalent in exponential growth and decay models, making them indispensable in finance, biology, and physics.

Challenges and Common Mistakes

While indices provide a powerful tool for simplifying expressions, you may encounter the following challenges.

1. One common mistake is forgetting the laws of indices when combining or simplifying terms. It’s crucial to revisit these laws regularly to build a strong foundation.
   
2. Another challenge arises when dealing with negative indices. Remembering that a negative exponent implies taking the reciprocal of the base raised to the positive exponent helps in avoiding errors.

Conclusion

Indices are a foundational concept in mathematics with widespread applications. By mastering the laws of indices, you will unlock the ability to simplify complex expressions and solve equations efficiently.

As you delve into the world of mathematics, a solid understanding of indices becomes a valuable asset, paving the way for success in more advanced mathematical concepts and real-world problem-solving.